Artigo
| Effects of solvent, base, and temperature in the optimisation of a new catalytic system for sonogashira cross-coupling using NCP pincer palladacycle |
|
Diego S. Rosa; Francine Antelo#; Toni J. Lopes; Neusa F. de Moura; Gilber R. Rosa*
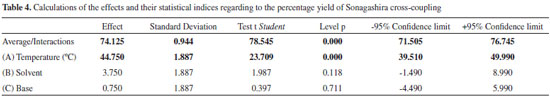
Escola de Química e Alimentos, Universidade Federal do Rio Grande - Campus Santo Antônio da Patrulha, Rua Barão do Cahy, 125, Cidade Alta, 95500-000 Santo Antônio da Patrulha - RS, Brasil Recebido em 18/12/2014 *e-mail: gilberrosa@furg.br The optimisation of a new catalyst system using NCP pincer palladacycle 1 was investigated using the experimental design technique. NCP pincer palladacycle 1 was previously investigated in Suzuki-Miyaura and Heck-Mizoroki cross-couplings and found to be a highly efficient catalyst precursor. In this study, the effects of the type of base (K3PO4 or DABCO), solvent (DMF or dioxane) and reaction temperature (130 or 150 ºC) in the second step on the reactional yield in Sonogashira cross-coupling were assessed using the two-factor design. The results showed that temperature is statistically significant in relation to the reaction yield. INTRODUCTION Sonogashira cross-coupling is an important C-C reaction between aryl- or vinyl halides and terminal acetylenes.1 The products of this reaction are key intermediates in the organic synthesis of natural products, pharmaceuticals and organic materials.2 In most cases, the classical experimental protocol involves using a palladium source such as [PdCl2(PPh3)2] or [Pd(PPh3)4] in the presence of Cu salts (Scheme 1).3
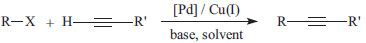 Scheme 1. Sonogashira cross-coupling
However, these traditional catalytic systems use large amounts of palladium (~ 5 mol%) and copper salts (~ 10 mol%), which prevent practical applications because of the difficulty of decontamination of the products and the high cost of the catalyst. Furthermore, the observed reactivity is of the following order: vinyl triflate ~ vinyl iodide > vinyl bromide > vinyl chloride > aryl iodide >> aryl bromide >> aryl chloride. Therefore, catalytic systems that promote the Sonogashira reaction with aryl chlorides are rare and fairly desired. More recently, copper-free catalytic systems promoted by palladacycles4 and efficient coupling of aryl chlorides even in aqueous media have been reported.5 Among these palladium sources, palladacycles have emerged as favourites particularly because of their unique properties, which include their facile synthesis, thermal stability and the possibility to readily modulate their steric- and electronic properties.6 They have CP, CN, CS, PCP, SCS, NCN and NCP types in their molecular structures, and they can have rings with 5 and 6 members.7 Our group has worked with NCP pincer palladacycle 1 and mentioned how Dupont's catalyst (Figure 1),8 which is a phosphinite palladacycle with an aliphatic backbone that can perform the cross-coupling of aryl chlorides with arylboronic acids (Suzuki-Miyaura reaction) or with n-butyl acrylates (Heck-Mizoroki reaction), results in notably good to excellent yields.7,9,10
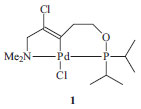 Figure 1. NCP pincer palladacycle (Dupont's catalyst)
However, the applicability of 1 in Sonogashira cross-coupling had not been tested. To develop a copper-free catalytic system, we attempted to use an experimental design tool. The factorial design is a useful analytical strategy, whose main application is the screening of relevant variables of a given system.11 After this screening process of the most significant variables, experiments are performed to allow refinement and a better understanding of the system under study.12 Thus, the tool can be adapted to develop a catalytic system. The present work aimed at studying and evaluating the effects of the base type (DABCO (1,4-diazabicyclo[2.2.2]octane) or K3PO4), type of solvent (DMF (N,N-dimethylformamide) or dioxane) and the reaction temperature (130 or 150 ºC) on the Sonogashira cross-coupling yield.
EXPERIMENTAL Experimental details All reactions were performed in an argon atmosphere in an oven-dried resealable Schlenk tube. All substrates, which included K3PO4, DABCO and DMF, were purchased from Acros. 1,4-Dioxane was purchased from Synth. The chemicals were used without further purification. The mass spectra were obtained using a GC/MS Shimadzu QP-5050 (EI, 70 eV). The gas chromatography analyses were performed using a Hewlett-Packard-5890 GC with an FID and 30 m capillary column with a dimethylpolysiloxane stationary phase. Palladacycle 1 was prepared as described in an early study.7 Typical experiment for Sonogashira cross-coupling An oven-dried resealable Schlenk flask was evacuated and back-filled with argon and charged with dried base (0.35 mmol), 4-bromoacetophenone (50 mg, 0.25 mmol), phenylacetylene (31 mg, 0.3 mmol) and NCP pincer 1 (0.2 mg, 5 × 10-4 mmol). The flask was evacuated and back-filled with argon, and 2 mL of solvent was subsequently added. The reaction mixture was stirred at 130 ºC or 150 ºC for 2 h (Scheme 2). The product was identified using GC/MS. The GC yield was taken as the average of two runs.
 Scheme 2. Sonogashira cross-coupling of phenylacetylene with 4-bromoacetophenone catalysed with 1
4-MeCOC6H4C≡CPh. GC-MS (IE, 70 eV) m/z (%): 220 (M+, 3), 205 (M+-Me, 23), 176 (38), 163 (5), 151 (15), 87 (26). Typical experiment for Suzuki-Miyaura cross-coupling An oven-dried resealable Schlenk flask was evacuated and back-filled with argon and charged with dried base (0.5 mmol), 2-bromomesitylene (50 mg, 0.25 mmol), 2-tolylboronic acid (51 mg, 0.37 mmol) and NCP pincer 1 (5 mg, 1.25 × 10-3 mmol). The flask was evacuated and back-filled with argon, and 2 mL of solvent was subsequently added. The reaction mixture was stirred at 130 ºC for 20 h (Scheme 3). The product was identified using GC/MS. The GC yield was taken as the average of two runs.
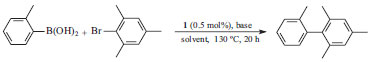 Scheme 3. Suzuki-Miyaura cross-coupling of 2-tolylboronic acid with 2-bromomesitylene catalysed with 1
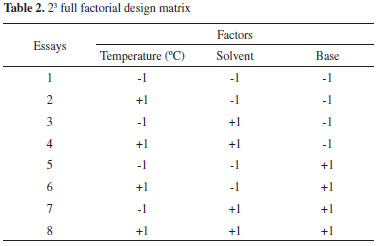
2,2',4,6-Tetramethylbiphenyl. GC-MS (IE, 70 eV) m/Z: 210 (M+), 195, 180, 179, 165, 152, 141, 128. Experimental design in Sonogashira cross-coupling reaction The experimental design is a tool that is used in many analysis processes, new formulations of systems operations and improvement of usual operation systems. The experimental design allows one to study the effects and relationship between one or more responses (output variable) and a set of factors (input variables), which are obtained as one of the advantages to reduce the number of tests. The yield of the Sonogashira cross-coupling reaction was evaluated according to the effect of the following variables: A) temperature, B) solvent, and C) base; their respective levels of variation are shown in Table 1. A full factorial 23 experimental design was used.
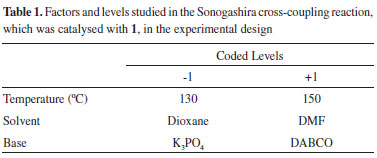
Table 2 shows the array of the full factorial 23 experimental design.
The results obtained during step 23 of the experimental array, which is obtained from an empirical statistical model, are used to predict the response variable in the studied process. The response variable is the reactional yield (%), which is considered a random variable y and distributed around a population average η(x1,x2) with a population variance σ2(x1,x2) (Equation 1):  where ε is the fluctuation around the middle values. For this statistical test, the model with only the main effects is used. Additionally, it was assumed that the deviations vary according to a normal distribution, i.e., for the full factorial planning, the average population η(x1,x2) can be represented by a linear combination of the variables x1 and x2 (Equation 2):  or through Equation 3: 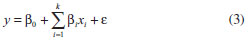 where β0 is the global average value of responses, and β1 and β2 are the population values of the linear effects of the main effects and the effect of the interaction per unit of x1 and x2. The statistical models that are tested to adjust the experimentally obtained values are evaluated according to the analysis of variance and coefficient of determination. Additionally, the location of levels of x1, x2, ...,xk that maximise the estimated response (predicted) is held. If this point exists, it is a set of x1, x2, ...,xk for which the partial derivatives are zero (Equation 4): 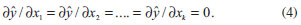 After the statistical analysis of coefficients, the effect of the factors and their interactions on the responses is analysed using the analysis of variance (ANOVA) and coefficient of determination (R2) to verify whether the model represents a degree of fit for the experimental data. These models were evaluated using the software Statistica 6.0®.
RESULTS AND DISCUSSION A full 23 factorial planning was used to evaluate the effect of the following variables: A) Temperature (ºC), B) Solvent, and C) Base with predefined coded levels on the percentage of Sonogashira cross-coupling yield. Table 3 shows the array of the complete planning and the 23 answers that were obtained for each test.
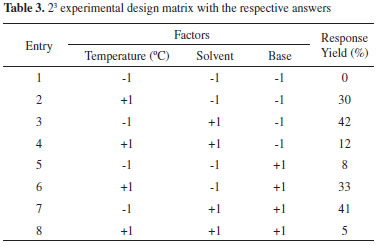
With the obtained results in Table 3, the effects of these three independent variables on the experimentally obtained responses and their respective statistical indices were analysed, as shown in Table 4.
In Table 4, note that the temperature effect is statistically significant for the level of confidence of 95% because the confidence interval (-95% to +95% confidence limit) does not contain the number zero, which indicates that the factor under consideration does not provide a null effect, i.e., the tested confidence level is not considered to be significant. Additionally, a coefficient of determination (R2) of 0.9929 was obtained. The algebraic sign of the observed effects is consistent with the knowledge of the phenomena involved. Because an increase in temperature leads to a higher percentage of income of the coupling reaction, the temperature is directly proportional with the yield. In addition, similar behaviours were observed for the calculated effects of the solvent factor (3.750) and base (0.750). Figures 2 and 3 show the contours that correspond to the response surface, which was generated using the empirical model for the temperature, solvent and base for a yield percent response of the reaction.
 Figure 2. Contours to the solvent and temperature factors
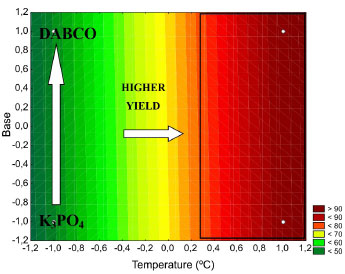 Figure 3. Contours to the base and temperature factors
Figures 2 and 3 show that with the variation in temperature of the reaction system, the percentage yield of the reaction sharply varies. In addition, the biggest reaction yields are obtained in the optimum region of 143-152 ºC (levels 0.3-1.2). For the tested bases and solvents, the system behaviour was hardly affected, and the base DABCO can be attached to the solvent DMF. In the empirical model for the percentage of income of the Sonogashira cross-coupling reaction, the studied levels are represented by Equation 5.  where Y is the yield of the reaction in percent (%), and T is the temperature of the reactional medium in ºC. The analysis of variance (ANOVA) shows that the value of the calculated F distribution (416.11) for the waste is related to regression, and the F distribution list is shown (5.9874 for 1 degree of freedom and 6 for 2 degrees of freedom). Thus, the decline is significant and can be used for prediction purposes. Additionally, the coefficient of determination (R2) of 0.9858 was obtained. All statistical indices show that the fitted model (Equation 5) describes well the experimental results. This result is contradictory to that of previous study for the Heck-Mizoroki coupling (Scheme 4).10 The reaction of 4-bromoanisole with n-butyl acrylate, which is catalysed by 1 with DABCO as the base and DMF as the solvent, gives the worst results. However, the effect on the reaction yield in both base and solvent is low.
 Scheme 4. Heck-Mizoroki cross-coupling catalysed with 1
K3PO4 (base) was evaluated for the Suzuki-Miyaura cross-coupling of different aryl halides with arylboronic acids that was catalysed with 1 (Scheme 5). Surprisingly, the base produced excellent yields in both reactions using DMF and using dioxane.7
 Scheme 5. Suzuki cross-coupling catalysed with 1
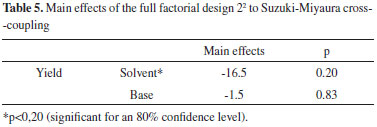
Additionally, a negative effect on the response performance was identified in another Suzuki-Miyaura cross-coupling investigation between 2-tolylboronic acid and 2-bromomesitylene using palladacycle 1 at constant temperature of 130 ºC but with KF and CsF bases, which changed the solvent from dioxane to DMF with an 80% confidence. Thus, when the solvent is changed to DMF, the reaction yield is reduced, i.e., from 56 to 45% with KF and from 60 to 38% with CsF. The base change for CsF to KF showed results similar to those of dioxane, which is an adverse effect on the yield (Table 5).
For the reaction temperature, we evaluated the catalytic system activation in Suzuki-Miyaura coupling with DMF and K3PO4. It was found that 1 required temperatures above 80 ºC for greater efficiency, and the optimum range was 100-130 ºC. In the cross-coupling of 4-chlorophenylboronic acid and iodobenzene at 25 ºC (room temperature), for a reaction time of 190 h, only 25% of the coupling product was obtained. When the catalyst concentration is increased by five times, the product concentration was only doubled (53%) under the identical reaction conditions, which correlates to the difficulty of palladacycle 1 in generating catalytically active Pd species at low temperatures. It is believed that the catalytically active species is the product of partial dechelation of NCP pincer 1; however, further investigation must be performed for such information.6 This fact also reinforces the positive response at high temperature (optimum region of 143-152 ºC) of the Sonogashira cross-coupling.
CONCLUSIONS In summary, the experimental design tool was used to evaluate the contribution of temperature, solvent and base in the development of a new catalytic system for Sonogashira cross-coupling using palladacycle 1. This evaluation permits the construction of a statistically significant model with 95% (correlation factor of 0.99), which describes the reaction yield as a function of these variables. Differences were found for other catalyst systems that have been optimised for Heck-Mizoroki and Suzuki-Miyaura cross-couplings using the same catalyst precursor. Such differences reside in the electronic properties of the substrates (phenylacetylene, n-butyl acrylate and arylboronic acid) and the steps of the catalytic cycle. However, 1 deserves special attention because it is one of the few phosphine-free palladium sources that promote high yields for both Heck and Suzuki cross-coupling reactions with aryl chlorides. In this work, 1 shows great potential to be applied in a copper-free catalytic system for Sonogashira cross-coupling of aryl bromides using low amounts of catalyst (0.2 mol%) in a short time (2 h). Our group continues working to enhance the catalytic system to be active with aryl chlorides and their applications in agrochemical fields.
ACKNOWLEDGEMENTS This work was supported by grants from CNPq and FAPERGS (Brazil).
REFERENCES 1. Negishi, E.; Handbook of Organopalladium Chemistry for Organic Synthesis, 1st ed., John Wiley & Sons: New York, 2002; Diederich, F; Meijere, A.; Metal-Catalyzed Cross-Coupling Reactions, 2nd ed., Wiley-VCHVerlag GmbH&Co: Weinheim, 2004; Karak, M.; Barbosa, L. C. A.; Hargaden, G. C.; RSC Adv. 2014, 4, 53442. 2. Chinchilla, R.; Nájera, C.; Chem. Rev. 2007, 107, 874; Chinchilla, R.; Nájera, C.; Chem. Soc. Rev. 2011, 40, 5084. DOI: http://dx.doi.org/10.1021/cr050992x PMID: 17305399 3. Kanda, K.; Koike, T.; Endo, K.; Shibata, T.; Chem. Commun. 2009, 1870; Severin, R.; Reimer, J.; Doye, S.; J. Org. Chem. 2010, 75, 3518. DOI: http://dx.doi.org/10.1039/b818904h 4. Blaszczyk, I.; Trzeciak, A. M.; Ziolkowski, J. J.; Catal. Lett. 2009, 133, 262; Consorti, C. S.; Flores, F. R.; Rominger, F.; Dupont, J.; Adv. Synth. Catal. 2006, 348, 133; Xu, C.; Lou, X. H.; Wang, Z. Q.; Fu, W. J.; Transition Met. Chem. 2012, 37, 519. DOI: http://dx.doi.org/10.1007/s10562-009-0181-y 5. Susanto, W.; Chu, C. Y.; Ang, W. J.; Chou, T. C.; Lo, L. C.; Lam, Y.; J. Org. Chem. 2012, 77, 2729. DOI: http://dx.doi.org/10.1021/jo202482h PMID: 22372634 6. Herrmann, W. A.; Bohm, V. P. W.; Reisinger, C. P.; J. Organomet. Chem. 1999, 576, 23; Dupont, J.; Pfeffer, M.; Spencer, J.; Eur. J. Inorg. Chem. 2001, 8, 1917; Bedford, R. B.; Chem. Commun. 2003, 15, 1787; Beletskaya, I. P.; Cheprakov, A. V.; J. Organomet. Chem. 2004, 689, 4055; Dupont, J.; Consorti, C. S.; Spencer, J.; Chem. Rev. 2005, 105, 2527; Dupont, J.; Pfeffer, M.; Palladacycles - Synthesis, Characterization and Applications, 1st ed., Wiley-VCH Verlag GmbH & Co.: Weinheim, 2008. DOI: http://dx.doi.org/10.1016/S0022-328X(98)01050-X 7. Rosa, G. R.; Ebeling, G.; Dupont, J.; Monteiro, A. L.; Synthesis 2003, 2894; Rosa, G. R.; Rosa, C. H.; Rominger, F.; Monteiro, A. L.; Dupont, J.; Inorg. Chim. Acta 2006, 359, 1947; Consorti, C. S.; Flores, F. R.; Dupont, J.; J. Am. Chem. Soc. 2005, 127, 12054; Gruber, A. S.; Zim, D.; Ebeling, G.; Monteiro, A. L.; Dupont, J.; Org. Lett. 2000, 2, 1287; Zim, D.; Gruber, A. S.; Ebeling, G.; Dupont, J.; Monteiro, A. L.; Org. Lett. 2000, 2, 2881. DOI: http://dx.doi.org/10.1055/s-2003-44352 8. Lopes, T. O.; da Silva Filho, D. A.; Lapis, A. A. M.; de Oliveira, H. C. B.; DaSilveira Neto, B. A.; J. Phys. Org. Chem. 2014, 27, 303; DaSilveira Neto, B. A.; Lapis, A. A. M.; Molecules 2009, 14, 1725. DOI: http://dx.doi.org/10.1002/poc.3234 9. Rosa, G. R.; Quim. Nova 2012, 35, 1052. DOI: http://dx.doi.org/10.1590/S0100-40422012000100019 10. Rosa, G. R.; Rosa, D. S.; RSC Adv. 2012, 2, 5080. DOI: http://dx.doi.org/10.1039/c2ra01261h 11. Montgomery, D. C.; Design and Analysis of Experiments, 1st ed., Wiley: New York, 1991. 12. Barros-Neto, B.; Scarminio, I. S.; Bruns, R. E.; Planejamento e otimização de experimentos, 1st ed., UNICAMP: Campinas, 1995; Rodrigues, M. I.; Iemma, A. F.; Planejamento de experimentos e otimização de processos, 1st ed., AMIC: Campinas, 2009. |
On-line version ISSN 1678-7064 Printed version ISSN 0100-4042
Qu�mica Nova
Publica��es da Sociedade Brasileira de Qu�mica
Caixa Postal: 26037
05513-970 S�o Paulo - SP
Tel/Fax: +55.11.3032.2299/+55.11.3814.3602
Free access